One of our goals in this chapter is to isolate issues about thinking procedurally. As a case in point, let us consider that, in evaluating nested call expressions, the interpreter is itself following a procedure.
To evaluate a call expression, Python will do the following:
- Evaluate the operator and operand subexpressions, then
- Apply the function that is the value of the operator subexpression to the arguments that are the values of the operand subexpressions.
Even this simple procedure illustrates some important points about processes in general. The first step dictates that in order to accomplish the evaluation process for a call expression we must first evaluate other expressions. Thus, the evaluation procedure is recursive in nature; that is, it includes, as one of its steps, the need to invoke the rule itself.
For example, evaluating
>>> sub(pow(2, add(1, 10)), pow(2, 5))
2016
requires that this evaluation procedure be applied four times. If we draw each expression that we evaluate, we can visualize the hierarchical structure of this process.
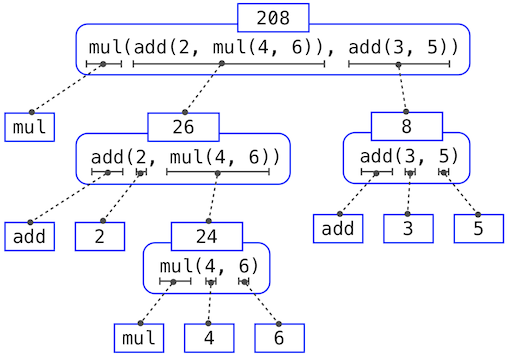
This illustration is called an expression tree. In computer science, trees conventionally grow from the top down. The objects at each point in a tree are called nodes; in this case, they are expressions paired with their values.
Evaluating its root, the full expression at the top, requires first evaluating the branches that are its subexpressions. The leaf expressions (that is, nodes with no branches stemming from them) represent either functions or numbers. The interior nodes have two parts: the call expression to which our evaluation rule is applied, and the result of that expression. Viewing evaluation in terms of this tree, we can imagine that the values of the operands percolate upward, starting from the terminal nodes and then combining at higher and higher levels.
Next, observe that the repeated application of the first step brings us to the point where we need to evaluate, not call expressions, but primitive expressions such as numerals (e.g., 2) and names (e.g., add). We take care of the primitive cases by stipulating that
- A numeral evaluates to the number it names,
- A name evaluates to the value associated with that name in the current environment.
Notice the important role of an environment in determining the meaning of the symbols in expressions. In Python, it is meaningless to speak of the value of an expression such as
>>> add(x, 1)
without specifying any information about the environment that would provide a meaning for the name x (or even for the name add). Environments provide the context in which evaluation takes place, which plays an important role in our understanding of program execution.
This evaluation procedure does not suffice to evaluate all Python code, only call expressions, numerals, and names. For instance, it does not handle assignment statements. Executing
>>> x = 3
does not return a value nor evaluate a function on some arguments, since the purpose of assignment is instead to bind a name to a value. In general, statements are not evaluated but executed; they do not produce a value but instead make some change. Each type of expression or statement has its own evaluation or execution procedure.
A pedantic note: when we say that "a numeral evaluates to a number," we actually mean that the Python interpreter evaluates a numeral to a number. It is the interpreter which endows meaning to the programming language. Given that the interpreter is a fixed program that always behaves consistently, we can say that numerals (and expressions) themselves evaluate to values in the context of Python programs.